There are a few more terms and definitions which relate to this topic. Some of them describe the same parameters as earlier, but from a slightly different approach.
Celestial Mechanics - More Terms

a : semimajor axis
For elliptical orbits, the semimajor axis is half the length of the longest line segment that can be drawn inside the ellipse. For hyperbolic orbits, the semimajor axis is the distance from the vertex to the nearest point on either branch.
e : eccentricity
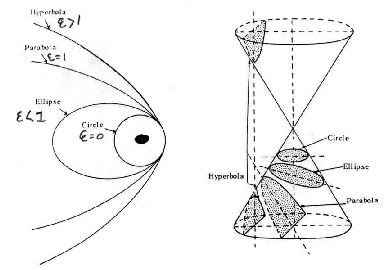
The orbit is a circle when e=0. The orbit is an ellipse when 0<e<1. The orbit is a parabola when e=1. The orbit is a hyperbola when e>1.
0mega: longitude of ascending node
The angle, subtended at the sun, measured in the ecliptic, from the Vernal Equinox to the ascending node. The ascending node is the point at which the orbit crosses the ecliptic having the Z component of its velocity in the direction of the North Ecliptic Pole.
[omega]: argument of perihelion
The angle, subtended at the sun, measured in the plane of the orbit in the direction of motion, from the ascending node to the orbit’s perihelion. (Most scientists use a lower-case omega for this quantity.)
T : time of perihelion passage
The time (e.g. a decimal calendar or Julian date) at which the orbiting object passes through the perihelion of its orbit.
Q : true anomaly
The angle, subtended at the sun, measured in the plane of the orbit in the direction of motion, from the perihelion to the current position of the orbiting object.
M : mean anomaly
The angle, subtended at the sun, measured in the plane of the orbit in the direction of motion, from the perihelion to the point at which the orbiting object would be found, if it moved constantly by its average angular velocity.
u : eccentric anomaly
The angle, subtended at the geometric center of the orbit, measured in the plane of the orbit in the direction of motion, from the perihelion to a point on the circle circumscribing the orbit, which point is found by extending a line perpendicular to the major axis through the current position of the orbiting object until it intersects the circumscribing circle.
Let’s spend a bit more time explaining how and why astronomers use eccentric anomaly.
When a body moves in an elliptical orbit, tracking its motion as a function of time is not as straightforward. The position of a body in elliptical motion is usually found by way of an intermediate property: the eccentric anomaly, E (or Ea in the later equation; refer to the diagram below).
Periapse is the general term for the point where an orbiting body is closest to the primary body.
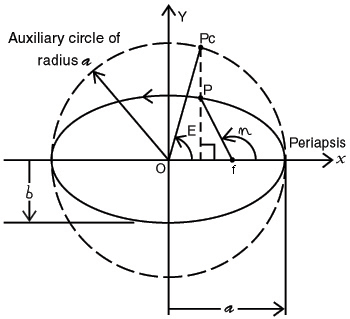
Given an ellipse with semi-minor axis b and semi-major axis a, a circle of radius a is superposed upon the ellipse. Assume that at time t, the body in elliptical motion is at point P. A vertical line is then drawn through P, up to where it intersects the superposed circle. Call this point Pc. The angle formed by drawing a line from Pc to the origin, to the body’s position at periapse defines the eccentric anomaly, Ea. Once Ea is found, calculating the true position of the body (point P) is quite simple.
Determining the eccentric anomaly requires solving Kepler’s Equation of Elliptical Motion:
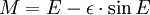
Back to elements of orbits.
Back to the science.
Sources: Transfer Orbits for Dummies! A Hillbilly Tutorial Found at: http://www.physicsforums.com/showthread.php?t=29524
Also: http://www.akiti.ca
